Mastering the Shift How to Convert Standard to Vertex Form Like a Pro

standard to vertex form are one of the most foundational topics in algebra and pre-calculus. From modeling projectile motion to solving standard to vertex form in business and engineering, they appear in countless real-world applications. But understanding a quadratic function goes beyond just plugging values into a formula—it’s about grasping the different ways the equation can be written and what each form reveals.

standard to vertex form skills in working with quadratics is knowing how to convert an equation from standard form to vertex form. Each form offers unique insights: standard form is great for calculating y-intercepts and simplifying expressions, while vertex standard to vertex form it easier to identify the turning point of the parabola. Being able to switch between them gives students and professionals a deeper, more versatile understanding of quadratic behavior.

In this article, we’ll take a comprehensive journey through this topic. We’ll explore the definitions and uses of each form, learn the step-by-step standard to vertex form the conversion, highlight common mistakes, and discuss the real-world relevance of vertex form. Whether you’re a student trying to ace a test or a teacher looking to explain this concept more clearly, this guide will help you master the shift from standard to vertex form—like a pro.

Understanding the Basics

What is a Quadratic Equation?

A quadratic equation is a second-degree polynomial equation in one variable, typically written in the form y=ax2+bx+cy = ax^2 + bx + cy=ax2+bx+c, where aaa, bbb, and ccc are constants and a≠0a \neq 0a=0. The defining feature of a quadratic is its squared variable, which gives the graph of the equation its signature U-shaped curve known as a parabola. The value of aaa determines whether the parabola opens upward (a>0a > 0a>0) or downward (a<0a < 0a<0).

Quadratic equations are all around us. They model the path of a basketball shot, calculate the area of a square plot, and even describe the profit curves in business scenarios. Understanding how these equations behave—and how to manipulate their structure—is key to unlocking their full potential. Most students are first introduced to quadratics in standard form, but this is just the beginning.

What is Standard Form?

The standard form of a quadratic equation is written as: y=ax2+bx+cy = ax^2 + bx + cy=ax2+bx+c

In this form:

• aaa is the coefficient that controls the width and direction of the parabola.
• bbb affects the position of the vertex along the x-axis.
• ccc is the y-intercept, showing where the graph crosses the y-axis.

Standard form is incredibly useful when performing algebraic operations like factoring or using the quadratic formula. It’s straightforward and ideal for solving roots, but it doesn’t immediately reveal the vertex—the highest or lowest point on the graph. For that, we need to use vertex form, which we’ll explore next.

What is Vertex Form?

The vertex form of a quadratic equation is: y=a(x−h)2+ky = a(x – h)^2 + ky=a(x−h)2+k

In this form, (h,k)(h, k)(h,k) represents the vertex of the parabola. This is where the graph reaches its minimum or maximum value, depending on the sign of aaa. The vertex form is incredibly useful for quickly graphing the parabola and identifying key features like the axis of symmetry, the vertex, and the direction in which the parabola opens.

Unlike the standard form, vertex form immediately tells us the turning point of the graph. If you’re analyzing motion, optimizing a function, or simply trying to sketch the graph, vertex form is a go-to. The question then becomes: how do we convert from standard to vertex form?

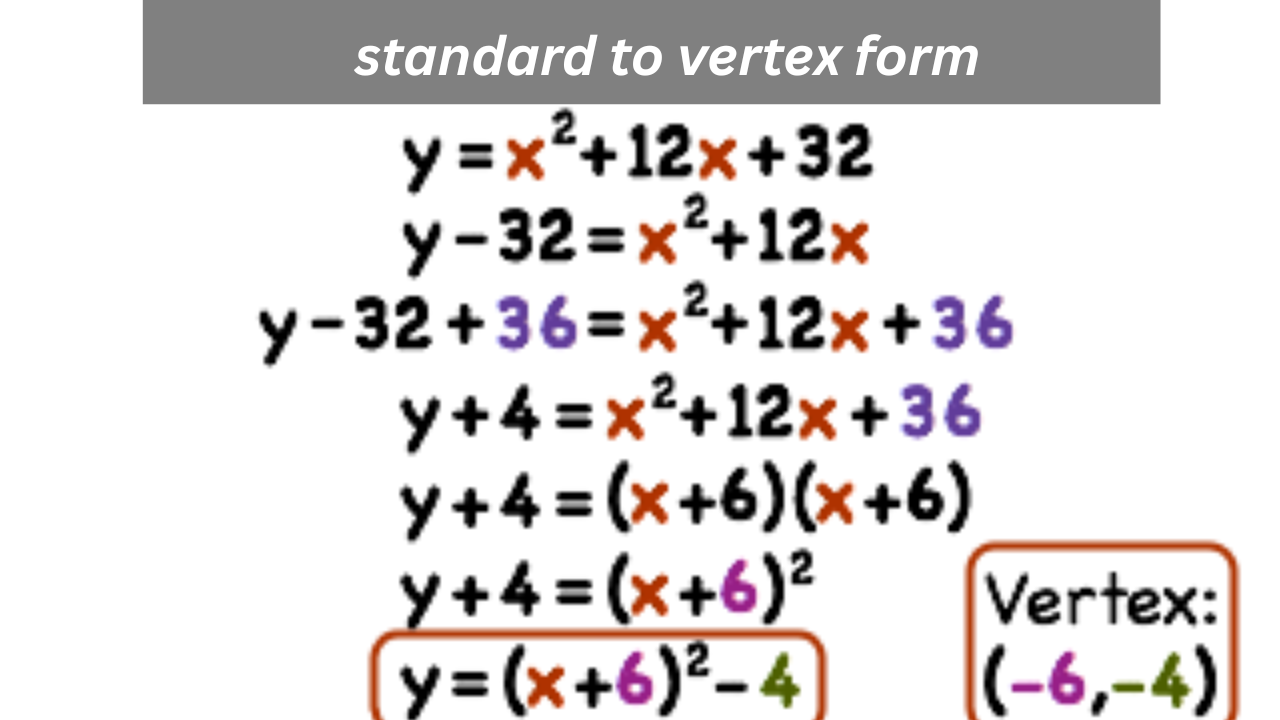
The Conversion Process

The Method: Completing the Square

To convert a quadratic equation from standard form to vertex form, we use a process called completing the square. This method rewrites a quadratic in a way that reveals a perfect square trinomial, allowing us to express the equation in vertex form. The key idea is to adjust the equation so that it takes the shape of a(x−h)2+ka(x – h)^2 + ka(x−h)2+k.

Here are the basic steps:

1. Start with y=ax2+bx+cy = ax^2 + bx + cy=ax2+bx+c.
2. If a≠1a \neq 1a=1, factor it out from the first two terms.
3. Take half of the coefficient of xxx, square it, and add it inside the expression.
4. Subtract the same value to maintain the equation’s balance.
5. Factor the perfect square trinomial.
6. Simplify the constants and write the final vertex form.

Detailed Walkthrough

Let’s convert y=2×2+8x+5y = 2x^2 + 8x + 5y=2×2+8x+5 into vertex form:

1. Factor out the 2 from the first two terms: y=2(x2+4x)+5y = 2(x^2 + 4x) + 5y=2(x2+4x)+5
2. Take half of 4 (which is 2), square it to get 4, and add and subtract inside the parentheses: y=2(x2+4x+4−4)+5y = 2(x^2 + 4x + 4 – 4) + 5y=2(x2+4x+4−4)+5
3. Group the perfect square trinomial and simplify: y=2((x+2)2−4)+5y = 2((x + 2)^2 – 4) + 5y=2((x+2)2−4)+5
4. Distribute and combine constants: y=2(x+2)2−8+5=2(x+2)2−3y = 2(x + 2)^2 – 8 + 5 = 2(x + 2)^2 – 3y=2(x+2)2−8+5=2(x+2)2−3

So, the vertex form is: y=2(x+2)2−3y = 2(x + 2)^2 – 3y=2(x+2)2−3

The vertex is (−2,−3)(-2, -3)(−2,−3).

Visualizing the Change

Graphical Comparison

When you convert a quadratic from standard to vertex form, you’re not changing the underlying function—you’re changing the way you see it. In standard form, the equation focuses on intercepts and general structure. In vertex form, it centers on the graph’s most critical point: the vertex.

Graphically, both forms produce the same parabola, but vertex form makes it easy to plot the vertex directly and then sketch the rest of the curve. This is especially useful for quick graphing without a calculator. The axis of symmetry becomes instantly clear (it’s x=hx = hx=h), and the direction of the curve is based on the sign of aaa.

Interactive Tools and Technology

Using tools like Desmos, GeoGebra, or graphing calculators can help students visualize the transition between forms. These platforms allow users to input both standard and vertex forms, instantly showing how the graph shifts but retains its overall shape. Seeing both representations side by side reinforces understanding and deepens mathematical intuition.

Real-World Applications

Vertex Form in Problem Solving

In many real-world problems, the vertex tells us the “best” outcome—maximum profit, highest point, lowest cost, etc. For example, in physics, the vertex of a parabola describing projectile motion gives the peak height of an object. In business, it can represent the point where revenue or profit is at its maximum.

Knowing how to convert to vertex form gives you direct access to this valuable information, allowing you to interpret and solve problems faster and more accurately.

Teaching and Learning Strategies

For educators, emphasizing both the why and the how of the conversion process can boost comprehension. Visual aids, hands-on graphing activities, and connecting math to real-life examples can make this concept stick. Encourage students to use both forms fluidly and understand the strengths of each. Practice problems, quizzes, and group challenges can reinforce skills and build confidence.

Conclusion

Converting a quadratic equation from standard to vertex form isn’t just a mechanical process—it’s a deeper way of understanding how quadratic functions behave. By completing the square, you unlock new insights into the graph, such as its turning point and symmetry. This powerful skill enhances your ability to solve real-world problems, graph efficiently, and approach algebra with a flexible mindset.

FAQs

You May Also Read: https://topbuzusa.com/factor-meals-menu/